?tgsyl

Developer Reference for Intel® oneAPI Math Kernel Library for C

Download PDF

ID 766684

Date 10/31/2024

Version

Public

Visible to Intel only — GUID: GUID-B2E62CEF-E067-47E3-A04B-4CC12F34C56F

View Details

?tgsyl

Solves the generalized Sylvester equation.

Syntax

lapack_int LAPACKE_stgsyl( int matrix_layout, char trans, lapack_int ijob, lapack_int m, lapack_int n, const float* a, lapack_int lda, const float* b, lapack_int ldb, float* c, lapack_int ldc, const float* d, lapack_int ldd, const float* e, lapack_int lde, float* f, lapack_int ldf, float* scale, float* dif );

lapack_int LAPACKE_dtgsyl( int matrix_layout, char trans, lapack_int ijob, lapack_int m, lapack_int n, const double* a, lapack_int lda, const double* b, lapack_int ldb, double* c, lapack_int ldc, const double* d, lapack_int ldd, const double* e, lapack_int lde, double* f, lapack_int ldf, double* scale, double* dif );

lapack_int LAPACKE_ctgsyl( int matrix_layout, char trans, lapack_int ijob, lapack_int m, lapack_int n, const lapack_complex_float* a, lapack_int lda, const lapack_complex_float* b, lapack_int ldb, lapack_complex_float* c, lapack_int ldc, const lapack_complex_float* d, lapack_int ldd, const lapack_complex_float* e, lapack_int lde, lapack_complex_float* f, lapack_int ldf, float* scale, float* dif );

lapack_int LAPACKE_ztgsyl( int matrix_layout, char trans, lapack_int ijob, lapack_int m, lapack_int n, const lapack_complex_double* a, lapack_int lda, const lapack_complex_double* b, lapack_int ldb, lapack_complex_double* c, lapack_int ldc, const lapack_complex_double* d, lapack_int ldd, const lapack_complex_double* e, lapack_int lde, lapack_complex_double* f, lapack_int ldf, double* scale, double* dif );

Include Files

mkl.h

Description

The routine solves the generalized Sylvester equation:

A*R-L*B = scale*C

D*R-L*E = scale*F

where R and L are unknown m-by-n matrices, (A, D), (B, E) and (C, F) are given matrix pairs of size m-by-m, n-by-n and m-by-n, respectively, with real/complex entries. (A, D) and (B, E) must be in generalized real-Schur/Schur canonical form, that is, A, B are upper quasi-triangular/triangular and D, E are upper triangular.

The solution (R, L) overwrites (C, F). The factor scale, 0≤scale≤1, is an output scaling factor chosen to avoid overflow.

In matrix notation the above equation is equivalent to the following: solve Z*x = scale*b, where Z is defined as

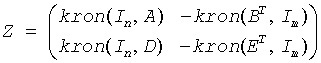
Here I_k is the identity matrix of size k and X^T is the transpose/conjugate-transpose of X. kron(X, Y) is the Kronecker product between the matrices X and Y.

If trans = 'T' (for real flavors), or trans = 'C' (for complex flavors), the routine ?tgsyl solves the transposed/conjugate-transposed system Z^T*y = scale*b, which is equivalent to solve for R and L in

A^T*R+D^T*L = scale*C

R*B^T+L*E^T = scale*(-F)

This case (trans = 'T' for stgsyl/dtgsyl or trans = 'C' for ctgsyl/ztgsyl) is used to compute an one-norm-based estimate of Dif[(A, D), (B, E)], the separation between the matrix pairs (A,D) and (B,E).

If ijob ≥ 1, ?tgsyl computes a Frobenius norm-based estimate of Dif[(A, D), (B,E)]. That is, the reciprocal of a lower bound on the reciprocal of the smallest singular value of Z. This is a level 3 BLAS algorithm.

Input Parameters

matrix_layout

Specifies whether matrix storage layout is row major (LAPACK_ROW_MAJOR) or column major (LAPACK_COL_MAJOR).

trans

Must be 'N', 'T', or 'C'.

If trans = 'N', solve the generalized Sylvester equation.

If trans = 'T', solve the 'transposed' system (for real flavors only).

If trans = 'C', solve the ' conjugate transposed' system (for complex flavors only).

ijob

Specifies what kind of functionality to be performed:

If ijob =0, solve the generalized Sylvester equation only;

If ijob =1, perform the functionality of ijob =0 and ijob =3;

If ijob =2, perform the functionality of ijob =0 and ijob =4;

If ijob =3, only an estimate of Dif[(A, D), (B, E)] is computed (look ahead strategy is used);

If ijob =4, only an estimate of Dif[(A, D), (B,E)] is computed (?gecon on sub-systems is used). If trans = 'T' or 'C', ijob is not referenced.

m

The order of the matrices A and D, and the row dimension of the matrices C, F, R and L.

n

The order of the matrices B and E, and the column dimension of the matrices C, F, R and L.

a, b, c, d, e, f

Arrays:

a (size max(1, lda*m)) contains the upper quasi-triangular (for real flavors) or upper triangular (for complex flavors) matrix A.

b (size max(1, ldb*n)) contains the upper quasi-triangular (for real flavors) or upper triangular (for complex flavors) matrix B.

c(size max(1, ldc*n) for column major layout and max(1, ldc*m) for row major layout) contains the right-hand-side of the first matrix equation in the generalized Sylvester equation (as defined by trans)

d (size max(1, ldd*m)) contains the upper triangular matrix D.

e (size max(1, lde*n)) contains the upper triangular matrix E.

f(size max(1, ldf*n) for column major layout and max(1, ldf*m) for row major layout) contains the right-hand-side of the second matrix equation in the generalized Sylvester equation (as defined by trans)

lda

The leading dimension of a; at least max(1, m).

ldb

The leading dimension of b; at least max(1, n).

ldc

The leading dimension of c; at least max(1, m) for column major layout and at least max(1, n) for row major layout .

ldd

The leading dimension of d; at least max(1, m).

lde

The leading dimension of e; at least max(1, n).

ldf

The leading dimension of f; at least max(1, m) for column major layout and at least max(1, n) for row major layout .

Output Parameters

c

If ijob=0, 1, or 2, overwritten by the solution R.

If ijob=3 or 4 and trans = 'N', c holds R, the solution achieved during the computation of the Dif-estimate.

f

If ijob=0, 1, or 2, overwritten by the solution L.

If ijob=3 or 4 and trans = 'N', f holds L, the solution achieved during the computation of the Dif-estimate.

dif

On exit, dif is the reciprocal of a lower bound of the reciprocal of the Dif-function, that is, dif is an upper bound of Dif[(A, D), (B, E)] = sigma_min(Z), where Z as defined in the description.

If ijob = 0, or trans = 'T' (for real flavors), or trans = 'C' (for complex flavors), dif is not touched.

scale

On exit, scale is the scaling factor in the generalized Sylvester equation.

If 0 < scale < 1, c and f hold the solutions R and L, respectively, to a slightly perturbed system but the input matrices A, B, D and E have not been changed.

If scale = 0, c and f hold the solutions R and L, respectively, to the homogeneous system with C = F = 0. Normally, scale = 1.

Return Values

This function returns a value info.

If info=0, the execution is successful.

If info = -i, the i-th parameter had an illegal value.

If info > 0, (A, D) and (B, E) have common or close eigenvalues.

Parent topic: Generalized Nonsymmetric Eigenvalue Problems: LAPACK Computational Routines

Select Your Language

Using Intel.com Search

Quick Links

Recent Searches

Advanced Search

Only search in

Developer Reference for Intel® oneAPI Math Kernel Library for C

?tgsyl

Syntax

Include Files

Description

Input Parameters

Output Parameters

Return Values