heevd

oneMKL - Data Parallel C++ Developer Reference

Download PDF

ID 772045

Date 7/13/2023

Version

Public

A newer version of this document is available. Customers should click here to go to the newest version.

Visible to Intel only — GUID: GUID-B5BC8D18-3883-4D1A-8244-D7652CA1CCBF

View Details

heevd

Computes all eigenvalues and, optionally, all eigenvectors of a complex Hermitian matrix using divide and conquer algorithm. This routine belongs to the oneapi::mkl::lapack namespace.

Description
API

Description

The routine computes all the eigenvalues, and optionally all the eigenvectors, of a complex Hermitian matrix A. In other words, it can compute the spectral factorization of A as: A = Z*Λ*ZH.

Here 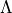is a real diagonal matrix whose diagonal elements are the eigenvalues 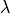_i, and Z is the (complex) unitary matrix whose columns are the eigenvectors z_i. Thus,

A*zi = λi*zi for i = 1, 2, ..., n.

If the eigenvectors are requested, then this routine uses a divide and conquer algorithm to compute eigenvalues and eigenvectors. However, if only eigenvalues are required, then it uses the Pal-Walker-Kahan variant of the QL or QR algorithm.

API

Syntax

namespace oneapi::mkl::lapack {
  void heevd(sycl::queue &queue,
  mkl::job jobz,
  mkl::uplo uplo,
  std::int64_t n,
  sycl::buffer<T> &a,
  std::int64_t lda,
  sycl::buffer<T> &w,
  sycl::buffer<T> &scratchpad,
  std::int64_t scratchpad_size)
}

heevd supports the following precision and devices.

T	Devices Supported
`std::complex<float>`	CPU and GPU
`std::complex<double>`	CPU and GPU

Input Parameters

queue

Device queue where calculations will be performed.

jobz

Must be job::novec or job::vec.

If jobz = job::novec, then only eigenvalues are computed.

If jobz = job::vec, then eigenvalues and eigenvectors are computed.

uplo

Must be uplo::upper or uplo::lower.

If uplo = job::upper, a stores the upper triangular part of A.

If uplo = job::lower, a stores the lower triangular part of A.

n

The order of the matrix A (0 ≤ n).

a

Buffer holding the array containing A, size (lda,*). The second dimension of a must be at least max(1, n).

lda

The leading dimension of a. Must be at least max(1,n).

scratchpad

Buffer holding scratchpad memory to be used by the routine for storing intermediate results.

scratchpad_size

Size of scratchpad memory as a number of floating point elements of type T. Size should not be less than the value returned by the heevd_scratchpad_size function.

Output Parameters

a: If jobz = job::vec, then on exit this buffer is overwritten by the unitary matrix Z which contains the eigenvectors of A.
w: Buffer holding array of size at least n. If info = 0, contains the eigenvalues of the matrix A in ascending order. See also info.

Exceptions

Exception	Description
`mkl::lapack::exception`	This exception is thrown when problems occur during calculations. You can obtain the info code of the problem using the info() method of the exception object: If `info = -i`, the `i`-th parameter had an illegal value. If `info = i`, and `jobz = job::novec`, then the algorithm failed to converge; `i` indicates the number of off-diagonal elements of an intermediate tridiagonal form which did not converge to zero. If `info = i`, and `jobz = job::vec`, then the algorithm failed to compute an eigenvalue while working on the submatrix lying in rows and columns `info/(n+1)` through `mod(info,n+1)`. If `info` is equal to the value passed as scratchpad size, and detail() returns non zero, then the passed scratchpad has an insufficient size, and the required size should not be less than the value returned by the detail() method of the exception object.

Exception

Description

mkl::lapack::exception

This exception is thrown when problems occur during calculations. You can obtain the info code of the problem using the info() method of the exception object:

If info = -i, the i-th parameter had an illegal value.

If info = i, and jobz = job::novec, then the algorithm failed to converge; i indicates the number of off-diagonal elements of an intermediate tridiagonal form which did not converge to zero.

If info = i, and jobz = job::vec, then the algorithm failed to compute an eigenvalue while working on the submatrix lying in rows and columns info/(n+1) through mod(info,n+1).

If info is equal to the value passed as scratchpad size, and detail() returns non zero, then the passed scratchpad has an insufficient size, and the required size should not be less than the value returned by the detail() method of the exception object.

Select Your Language

Using Intel.com Search

Quick Links

Recent Searches

Advanced Search

Only search in

oneMKL - Data Parallel C++ Developer Reference

heevd

Description

API